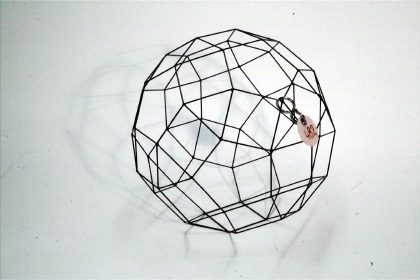
Rhombicosidodecahedron
Model 482
Description
(12+20+30)-plane polygon with 60 vertices, polar figure of the deltoidal hexecontahedron.
Additions
This model depicts the rhombicosidodecahedron, one of 13 Archimedian solids. The Archimedian solids belong to the class of semi-regular solids and were discovered by Archimedes in the third century BC. The completeness of this lists was proved in the year 1619 by Johannes Kepler.
Note that snub hexahedra and snub dodecahedra occur in two mirrored versions respectively, which is why some literature speaks of 15 Archimedian solids. A solid is called semi-regular if all its faces consist of regular polygons and if every vertex of the polyhedron can be mapped to any other vertex by the solid's symmetry operations.
The Archimedian solids can be obtained from the 5 Platonic solids by cutting off vertices and/or edges to create new faces. This means that Archimedian solids can only have 2 or 3 different polygons as faces. The rhombicosidodecahedron for example consists of 12 pentagons, 30 squares, and 20 triangles.
Archimedian solids have the following properties:
- All edges have the same length.
- The faces are regular polygons.
- The solids are dual to the Catalinian solids..
- The faces occuring at one single vertex determine the Archimedian solid uniquely.
- Every Archimedian solid has a circumscribed sphere and a midsphere, but no inscribed sphere.
Showcase of this model is Case number 21
References
Heesch, H.. Comm.Math.Helv, 6, n=4, Case 4b, fig.15.
Hess, E.(1883). Kugelteilung, Teubner, mit Figuren, §36, fig. 20.
Roman(1987). Reguläre und halbreguläre Polyeder, VEB Deutscher Verlag der Wissenschaften, p. 47 f..