The types of the development of a space curve at a small scale
Model 167
Description
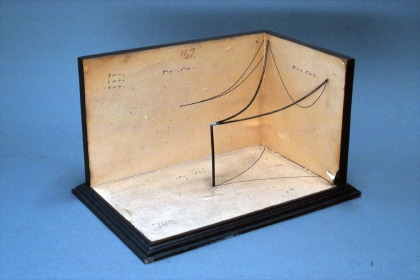
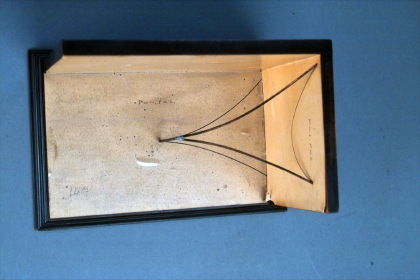
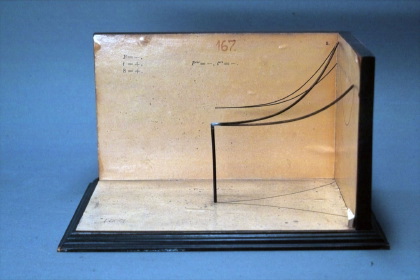
Models 163-170: The types of the development of a space curve at a small scale. With projections to the main planes.
Additions
In the year 1879, Christian Wiener commissioned eight models illustrating space curves. Space curves are maps \[f : \mathrm{I} \to \mathbb{R}, s \mapsto (f_1(s),f_2(s),f_3(s)), \] where $\mathrm{I}$ is a real interval, and $f$ has derivatives of all orders.
Space curves are characterized by their curvature and their torsion. The curvature gives a measure of how much the curve deviates from being a straight line, the torsion determines how much the behavior of the curve differs from a plane (twisting).
The shape of the curve can be described by a point progressing along the curve and either maintaining its direction of motion (+) or reversing it ($-$), analogously for the turning angles of tangent and osculating plane. There are precisely eight combinations, each of them illustrated by a model. Furthermore, the projections onto the tangential plane ($z=0$), the rectifying plane ($y=0$) and the normal plane ($x=0$) are displayed.
Showcase of this model is Case number 13
References
Schilling, Martin(Hrg.): Catalog mathematischer Modelle, Leipzig(Verlag von Martin Schilling) 1911, 7.Auflage, No.134-141. p. 131.
Z.f. Math. und Ph. Vol. 25, Die Abhängigkeit der Rückkehrelemente der Projektionen.
Fischer, Gerd(Hrg.): Fotoband: Mathematische Modelle / Mathematical Models, mit 132 Fotografien, Braunschweig/Wiesbaden (Vieweg) 1986, picture(s) 57-64. .
Fischer, Gerd(Hrg.): Mathematical Models, Commentary, Braunschweig/Wiesbaden(Vieweg) 1986. .
Separataband M1 im Mathematischen Institut p. 207.
Saurel(1905). On the Singularities of Tortuous Curves. Annals of Math..