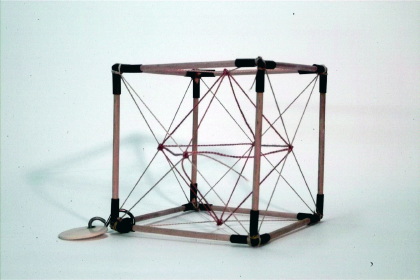
Cube and inscribed octahedron
Model 410
Description
Cube and inscribed octahedron. Fiber model.
Additions
This model shows a cube with an inscribed octahedron. The construction of the octahedron works as follows:
Using the intersection points of the diagonals, the centers of the faces on the cube can be constructed (here as wire). The centers of two adjacent faces get connected respectively (here as red thread). The emerging object is an octahedron that has the same center as the cube.
Performing the same steps with the octahedron yields a cube that is similar to the original cube. This is why cube and octahedron are called dual to each other.
The following duality relations hold for Platonic solids:
- hexahedron (cube) $\leftrightarrow$ octahedron
- dodecahedron $\leftrightarrow$ icosahedron
- tetrahedron $\leftrightarrow$ tetrahedron (the tetrahedron is dual to itself)
The number of faces of one polyhedron equals the number of vertices on its dual.
Showcase of this model is Case number 20
References
Separataband M4 im Mathematischen Institut p. 239.
Separataband M5 im Mathematischen Institut p. 41, 46-47.
Separataband M5 im Mathematischen Institut p. 5.
Hilbert, D.; Cohn-Vossen(1932). Anschauliche Geometrie, Springer-Verlag, Berlin, p. 79 f.. Online version